Whackamolean Epidemiology

Listening to the Prime Minister’s speeches on the radio, one hears irregular thuds in the background, as if a workman in a distant room was testing the heft of a sledgehammer. The thuds are in fact the Prime Minister thumping the desk, dispatch box, or lectern, a displacement activity occasioned by his spectacular inability to hit a single covid nail on the head, let alone whack even a solitary virus. One fears the thuds will become louder and more frequent as time goes by, and start to drown out the words, until one day all speeches come to be the cacophony of a multitude of workmen beating a devil’s tattoos with their hammers as they demolish the stony guardians of ancient British liberties one by one, until we become, as Mainwaring almost said, a nation of automatons led by a lunatic who looks like a clown.
By a round the houses route, the details of which we can let slip, that brings Dr No back to herd immunity. Once merely the heartthrob of vaccinologists, herd immunity entered the lexicon of covid early in the pandemic, and enjoyed a brief sparkle in the sunlight, before its prospects suffered a sharp decline, and it became seen as distinctly distasteful, on a par with fumigating a sewer to exterminate the rats, and it all but disappeared from view.
Disappeared, yes, but it didn’t go away, for it has always been there, in the background, as the whack that could end a thousand moles. Herd immunity, to recap, is the notion that once enough individuals in a population are immune to an infection, then any epidemic of that infection either will not happen, or if it is already in play, will fizzle out, like a damp sparkler. That is the primary purpose of herd immunity, to prevent or stop an epidemic, but a secondary consequence, which gives it its never quite right sounding name, is that it also affords protection for those who, for whatever reason, are not immune to the infection, by removing the infection from general circulation.
Herd immunity gives rise to the herd immunity threshold, which is the magic number, or rather percentage of the population, that need to be immune to stop an infection getting a hold, or, if it already has a hold, to cause an epidemic to fizzle out. This happens when the R number, the number of new cases infected by each individual case, drops below one. We need to know what percentage of immune people we need to keep the R number below one.
In the historical, conventional setting of infectious disease control through vaccination, where the entire pre-vaccination population is susceptible to infection, this is remarkably easy to determine. Let us say that R0, the so-called basic reproduction number, which is the number of new cases infected by each individual case in a fully susceptible population, is three. This is what gives us the epidemic: first one case, then three, then nine, then 27, and so on, as the epidemic spreads exponentially.
Now let us focus on that first case, and the three subsequent infections (all the other subsequent infections follow the same pattern): how many of the three need to be immune to get the R number down to one? The answer is two: if two of the three are immune, then only one gets infected, and so the R number becomes one.
More generally, the number that need to be immune is R0 – 1: if R0 is 3, as above, then 2 need to be immune, if R0 is 4, then 3 need to be immune, and so on. To convert that number into a rate (percentage), and so the herd immunity threshold, which is the point at which enough people are immune to bring R down to one, all we need to do is divide R0 – 1 by R0 and so the general formula becomes herd immunity threshold = (R0 – 1) / R0. For some reason – most probably obfuckstration — this is more commonly rearranged to a somewhat baffling 1 – 1 / R0, which were it not for operator precedence looks like zero. The rather inelegant Figure 1 shows how herd immunity works in practice.
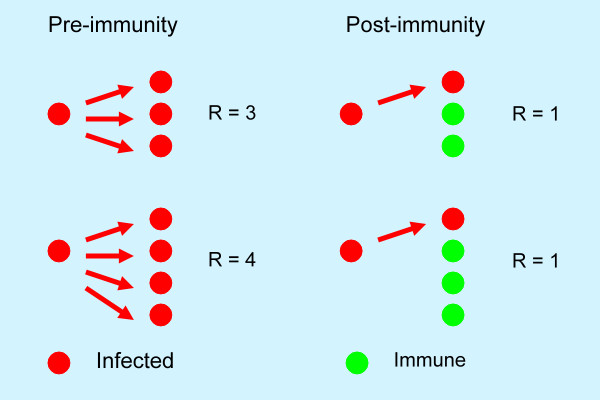
Figure 1: effect of immunity on R number: all individuals susceptible with R0 nunber on left, number (in green) needed to be immune to get R down to 1 on right.
Now, for covid–19, with a presumed best estimate of R0 of 2.5 (not shown on Figure 1, as it would involve quirky half circles), the herd immunity threshold works out at 1.5 /2.5 = 0.6, or 60% as a percentage, and this is what gave herd immunity its bad name. By simple back of the envelope sums, if we need 60% of the population to be infected and so become immune to gain herd immunity, then in the UK alone, even with a low mortality rate, that means hundreds of thousands of deaths. This is the point back in March when, to reuse a phrase from a previous post, Fergie farted, [and] the cabinet panicked.
But there is a problem with all this. The threshold has been determined using mass vaccination assumptions: a fully 100% susceptible population, with a fixed R0, managed by a mass and so random vaccination programme. This is not what happens in the real world, when immunity is gained not by mass vaccination, but by natural spread of the disease, because the natural spread of the disease naturally selects the most susceptible.
This is not some sort of Darwinian dystopia. Let’s go back to our sparkler. Let us imagine that, unknown to the sparkler marshals, it is a duff batch, and the bottom half of the sparkler lacks flammability. The sparkler marshals, guided by mass vaccination theory, believe at least 60% of the sparkler must be damp to extinguish the sparkler. But, in the real world, only 50% needs to be damp, so long as it is the right 50%, to extinguish the sparkler. And it might even be less, considerably less. If, for example, the sparkler is always lit by the tip, maybe only the top 10% needs to be damp to mean the sparkler never gets going in the first place.
This analogy is somewhat stretched — for a start, a single sparkler burns linearly, so to make the analogy work better we would have to imagine a box setup where each sparkler ignites a number of other sparklers — but it does make the point that when the mass vaccination assumptions don’t apply things can be different, perhaps very different. Let us go back to Figure 1. As presented, it shows herd immunity gained through mass vaccination. For the top row, two out of three, 67%, need to be vaccinated, and for the bottom row, three out of four, 75%, need to be vaccinated.
Now let us imagine that instead of using vaccination, we allow natural spread of infection and so selection of individuals to give rise to immunity. The first thing we encounter is that some people are simply not susceptible to infection. It might be they have partial cross immunity from another coronavirus, or are generally fit, can naturally resist infection. Alternatively, they may become infected, but not spreaders. In both cases, they behave, that is, they interact with others, as if they are immune. Let us say that for each row, one of the individuals on the right marked as immune is in fact one of these ‘as if’ immune people. For the top row, that halves the number that need to gain immunity through infection, from two to one, or from 67% to 33%. The drop in the second row, from 75% to 50%, is not quite so dramatic, but is still fairly potent.
The second thing is the infection naturally selects the most susceptible, so, at the risk of stating a tautology, it is the susceptible that get infected. Yes, some will die, but that is always going to be the case; the key point is that most will recover, and in the process the natural selection of the most susceptible has by it’s nature efficiently selected the most susceptible, and so gives the most at risk immunity. The virus is simply more efficient than a random vaccine, because it is more selective: it targets those who need immunity the most.
A third consideration is the crude R0 number, which is a very basic across the board number, is in the real world neither basic nor across the board, and instead varies, typically downwards, because R0 is the ‘worst case scenario’. Even a soft lockdown, as in Sweden, will lower R0 somewhat, and lowering the R number always lowers the herd immunity threshold. Any such lowering cause by so called non-pharmaceutical measures acting to lower R will be in addition to the lowering of the threshold brought about by the presence of ‘as if’ immune individuals in the population.
Dr No is only too aware this post is far from rigorous in its treatment of its subject, and it is offered chiefly as a suggestion that perhaps we need to reconsider, perhaps radically, our assessment of what the herd immunity threshold is in the real world, rather than relying on a rate based on mass vaccination assumptions. For a more rigorous investigation, with equations to make the eyes pop, and jargon enough to cause water on the brain, consider this well titled advanced treatment of the subject. It includes the concept of ‘effective herd immunity’, as measured by seroprevalence, that is, natural immunity gained through infection, which is what Dr No has been talking about, and comes to broadly similar conclusions. The authors sum up [emphasis added]:
“Put simply, an effective herd immunity — that works hand-in-hand appropriate public health and local lockdown measures — requires less than 20% seroprevalence. This seroprevalence has already been reached in many countries and is sufficient to preclude a traumatic second wave, even under pessimistic assumptions about loss of humoral immunity endowed by antibodies.”
Dr No suggests it is time we stopped dismissing herd immunity as a ghastly Darwinian dystopia, and instead give proper consideration to its potential to help us move on from the ghastly state-imposed dystopia in which we currently find ourselves.

Very interesting discussion. I think you have a typo in para 7. R0 – (R0-1) always equals 1.
John – thanks. You are also right about the typo. The biggest problem I had was getting the subscript zeros – same problem here in the comments, where it is even less fixable, and I foolishly took my eye off the content. Have corrected the post from
“More generally, the number that need to be immune is R0 – (R0 – 1): if R0 is 3, as above, then 2 need to be immune, if R0 is 4, then 3 need to be immune, and so on.”
to
“More generally, the number that need to be immune is R0 – 1: if R0 is 3, as above, then 2 need to be immune, if R0 is 4, then 3 need to be immune, and so on.”
Have you seen Nic Lewis’s (independent climate scientist, maths/physics background) contributions to the topic?
https://judithcurry.com/2020/07/27/why-herd-immunity-to-covid-19-is-reached-much-earlier-than-thought-update/
How did “herd immunity” become a toxic phrase? Is it as simple as being the subject of propaganda from the Guardian, the Beeb, and the like?
I notice that “algorithm” is beginning to attract equivalent status, becoming a scare-word.
There seem to me to be two routes to ending the great pandemic scare. The one that might involve fewer deaths would be to hang politicians, mathematical modellers, members of Sage, and journalists in large enough numbers to encourage the others. We could look upon them as sacrifices for the greater good.
Tish – I have, and also took note of the fact he has been rather roundly taken to task for dabbling outside his area of expertise (‘independent’ climate scientist is for some a euphemism for climate change denier, and so he may be being attacked for this as much as the veracity or otherwise of his covid claims). That said, given what I have written in this post, we are certainly on the same hymn sheet, as are Friston, Costello and Pillay who co-authored the ‘well titled’ Dark Matter paper linked to in the post. The key point, expressed in its various ways, is that a herd immunity threshold calculated on a fully susceptible population, mass vaccination programme basis is too simplistic, and ignores other factors that come into play in the real world. Friston by the way is the same Karl Friston who gave us the memorable ‘all that can be burned, has been burned’ quote that Dr No used for this May 2020 post:
https://dr-no.co.uk/2020/05/15/all-that-can-be-burned-has-been-burned/
There is absolutely no doubt that if effective herd immunity is reached at lower levels (say around 20%, as measured by seroprevalence, so these are the ones who have gained immunity, assuming they are immune, through natural exposure, given there is no vaccine) then we are in a much better place than many think we are, because many countries are already approaching such levels.
The trouble is we now have a mighty political/industrial/commercial/WHO juggernaut so large it can be seen from a distant galaxy rushing along crushing everything in its path, including the far too mundane possibility that we might just be getting somewhere by in effect muddling through.
dearieme – Herd Immunity was until recently a bit of a niche and at the same time Marmite phrase, beloved by vaccinologists, but disliked by the caring and sharing so much it hurts brigade, as in their caring and sharing world, anything that suggests humans are herds is prima facie demeaning etc, demonstrating they missed the point that it was being used in a technical way to describe an effect, not people. That said, it has never really worked for Dr No, a nice try but no cigar phrase, because it borrows from herd safety behaviour in animals, and doesn’t quite fully make the animal behaviour to human immunology jump successfully.
It shot to fame and then toxicity most likely because of timing. It’s brief vogue was just before Fergie produced his infamous 500,000 dead dossier of despair, so the two became inextricably linked. One moment we had herd immunity as the way forward, then the next a dossier saying righty-ho, that’ll be 500,00 dead then. Anything that leaves 500,000 dead by the roadside has got to be toxic etc etc, and once the toxic label got attached, it stuck.
Algorithms, models and equations are cousins, but are not interchangeable, as sometimes happens, often by journalists, who should know better, but are prone to get seduced by sciencey sounding words. The Ofqual exam grading equation got dubbed an algorithm by the media, and as the exam debacle unfolded, so algorithm became a dirty word.
Let’s hang them all – you have the mind of Dick the Butcher from Henry VI, Part 2!
“The Ofqual exam grading equation got dubbed an algorithm by the media, and as the exam debacle unfolded, so algorithm became a dirty word.”
Long before that, the use of ‘algorithm’ with reference to targeted advertising by Amazon, Google et al. had already given algorithm a sinister undertone.
djc – Indeed, very true, and and even before that the dreaded ‘care pathways’ were seen by many as patient processing algorithms that turned patients into sausages. Woe betide any square sausages that got jammed in the machine…
Government Vaccine Roll-out consultation officially closes Sept 18, but will receive comments after this:
https://www.gov.uk/government/consultations/distributing-vaccines-and-treatments-for-covid-19-and-flu/consultation-document-changes-to-human-medicine-regulations-to-support-the-rollout-of-covid-19-vaccines